Batch 2 - Class 18 - Solid Geometry
Pre-class problems:
Crown and Anchor - You have a board marked with 6 symbols (crown, anchor, diamond, spade, club and heart - but we will use 1-6 numbers), and dice marked with same symbols. You place a bet at any one square, and then roll three dice. If one dice comes up with the same symbol where you placed the bet, you get twice the amount; if two dice turn up, you get three times; and if 3 dice turn up, you get 4 times.
- If you play with two dice (double money on one hit, triple on 2 hits), what would you expect to win or lose?
- If you play with three dice (regular game), what would you expect to win or lose?
- Do the odds change if you bet on two numbers simultaneously? (either in two or three dice game)
- Is there a strategy a player can adopt to always win on a particular day?
- What happens if you have a fourth dice (and 5x payout if all four turn up the bet symbol)?
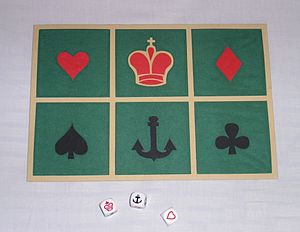
Answer: 92.1% probability of take back on any particular game, so house has an edge. If you bet on two numbers simultaneously, the average take back is still 92.1% (Its like two people playing the game on same board). To always win, you can use the strategy of doubling the bet on every successive game. With four dice, the odds rise to 1.18, so the house loses!
- Homework trivia: why do we say "curiosity killed the cat"
Attendance Rhea, Anisha, Muskaan, Tishyaa, Aastha, Hriday
Class puzzles
- (MartinShCol - 6.2) You want to construct a rigid wire skeleton of one-inch cube by using 12 one-inch wire segments for the cube's 12 edges, and soldering them at eight corners. If you were allowed to cut down the number of soldering points by using one or more longer wires that you can bend at sharp corners, what is the minimum number of points of soldering.Answer: Still eight. Because an odd number of edges meet at each corner of the cube, so each corner still requires soldering(MartinShCol - 6.4) Construct a 6x6x6 cube with 27 bricks that are each 1x2x4 unitsAnswer: Can't do this. Think of 6x6x6 cube made of 27 2x2 cubes with alternate black and white colors. Since there are unequal number of black and white squares, and no matter how you place a brick half of its 6 cubes are black and half are white, you can't construct the larger cube.(MartinShCol - 6.7) A carpenter wishes to cut a wooden cube, three inches to a side, into 27 one-inch cubes. What is the minimum number of cuts required to do so? What are the minimum number of cuts for a 4 inch cube (64 one-inch cubes)?Answer: 6 for three inch cube; 6 also for 4x4x4 cube.(MartinShCol - 6.8) Design a cork so that it fits a square, a circular and a triangular hole snugly.Answer: Take a cylindrical cork standing on a face, and cut a triangle as you look at it. The base provides the circular fit, the cylinder's cross section provides square, and the triangle is the third.
- (MartinShCol - 6.10) Make a single cut through a cube to get a hexagonal surfaceAnswer: Take mid points of six sides and cut through them
Homework Game: Given a convex n-gon, the players take turns drawing diagonals that do not intersect those diagonals that have already been drawn. The player unable to draw a diagonal loses. What if there are multiple such n-gons and you play overall?
- Answer: Odd sided n-gon - second guy wins; Even sided n-gon - first guy wins. Invariant is that if there are odd number of even sided n-gons at any stage, then first guy wins, else second
References:
The Colossal Book of Short Puzzles and Problems, by Martin Gardner